The apparently infinite value of bitcoin has been expressed in a proposed mathematical situation before.
If you’ve fallen down the Bitcoin rabbit hole even a foot, you’ve probably seen references to the idea of “everything there is, divided by 21 million.” Originally posited by Knut Svanholm, Ioni Appelberg and Guy Swann in a YouTube video with the same title, the concept reflects the seemingly infinite potential value of Bitcoin. As it continues to overtake various asset classes like gold and spread into new markets, the total value of the Bitcoin market appears to be only constrained by the absolute maximum of 21 million circulating bitcoin.
When a financial endeavor promises, in theory, to yield infinite (divided by 21 million) returns, it’s easy to get caught up in the hype. People have even taken out mortgages to buy bitcoin. In the face of infinitely high returns, this isn’t irrational. Why would you not pay anything and everything for the chance to make your life infinitely better? However, the St. Petersburg game, a thought experiment in economics that can trace its roots all the way back to 1713, may offer some insights into the hype surrounding the potential value of bitcoin.
The St. Petersburg game means a lot more than the Bitcoin Bowl in St. Petersburg, Florida. Originally published in Papers of the Imperial Academy of Sciences in Petersburg by Daniel Bernoulli, the St. Petersburg paradox is a thought experiment that pushes traditional behavioral economics to the test. Imagine that you’re asked to pay some amount of money to participate in a bet. In the bet, a fair coin is tossed until it shows heads. When it eventually shows heads, you get paid two dollars multiplied by the number of times it was tossed. If it lands on heads on the first toss, you get paid $2. If it lands on tails on the first toss and heads on the second toss, you get paid $4. If it lands on heads on the third toss, $8, and so on and so forth. How much should you be willing to pay to play this game?
Pure economic decision theory would tell you to pay any finite amount. You should sell your car, take out a third mortgage on your home, and contact every loan shark in your address book just for the opportunity to play the St. Petersburg game. Let’s look at why an ideally rational person could end up at such an irrational-seeming conclusion.
For a person seeking to maximize expected value, the traditional approach to decision theory is to take the amount of value in a payout and multiply it by the probability of that payout happening. The probability of the coin landing on heads in the first toss is 50 percent and the payout is $2. Multiplying these together gives us an expected value of $1. The probability of the coin landing on heads in the second toss is 25 percent and the payout is $4, giving us an expected value of $1 again. You can calculate the expected value for any number of tosses n and it will always yield $1.
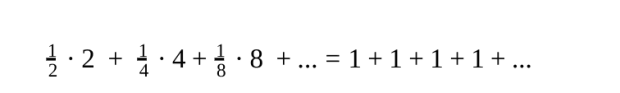
What are the odds that the coin lands on tails a million times before finally landing on heads? Really really low (around 10−301030), but the amount you stand to gain is $2 million. To find the maximum amount of money that you should be willing to play this game, you can add the sum of expected values for all potential outcomes. As the number of tails tosses in a row increases, the probability gets incredibly small, but it is never zero. As such, we should take the sum of an infinite set.
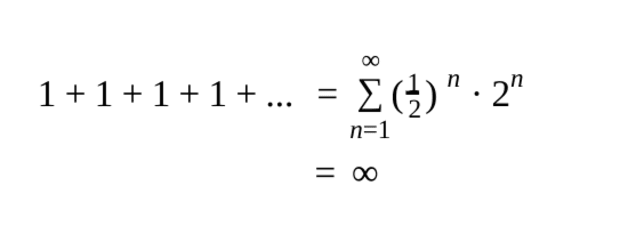
As you can see, this gives us a total expected value of infinity for the game. With a potential payout approaching infinity like this, you could pay any finite amount of money to play the game and still maximize your expected value.
This recommendation has confused economists for centuries. How could it possibly be reasonable to pay millions or even billions of dollars for an infinitesimally small chance at a net-positive payoff? There’s no conclusive answer so far, but decreasing marginal utility is a long-standing suggestion for why you shouldn’t pay a ludicrous amount. Decreasing marginal returns is the idea that at some point, once all your needs are met, additional dollars are worth less to you than before. Vitalik Buterin explained in this article that a gallon of water a day is worth a lot more when you have none than when you already have 99. Similarly, in the St. Petersburg game, it may seem justified to risk losing a billion dollars to gain three billion, but if you already have a billion dollars, how much can an extra billion really do for you? If you lose, the difference in lifestyle between zero dollars and a billion dollars is much more extreme than the difference in lifestyle between a billion dollars and three billion dollars. This is true for more realistic sums of money as well.
Now what does all this have to do with Bitcoin? If Bitcoin can truly represent the total value of the entire economy — past, present, and future — then it’s the closest thing we’ve ever seen to a real-life St. Petersburg game. Like the St. Petersburg game, the payout itself would be finite (bitcoin will probably always have a quantifiable value attached to it), but its potential approaches infinity.
In one sense, this highlights the asymmetric risk-reward tradeoff of investing in bitcoin. You can only lose what you put in, but the potential reward is much, much higher. In another sense, this thought experiment recommends putting everything you can into bitcoin, without paying any mind to your potential losses. The only thing to keep in mind when you’re deciding whether or not to put an extra $100 of your fiat currency into bitcoin is how you could use that $100 right now. If the money is the difference between paying your utilities or having your water cut off, it’s probably smarter to hold onto it. After all, even the best scenario for bitcoin won’t add as much happiness to your life as forgoing showers for a month would take away. But if you’ve paid off all your credit cards and the rent check is in the mail, then know the extra dollars you put into bitcoin are endorsed by economic theory over the past few hundred years.
This is a guest post by Will Henson. Opinions expressed are entirely their own and do not necessarily reflect those of BTC, Inc. or Bitcoin Magazine.
via bitcoinmagazine.com


